Prepare to excel in your Chapter 3 Geometry assessment with our comprehensive guide, providing you with chapter 3 chapter test geometry answers and expert insights to master the fundamental concepts and problem-solving techniques.
Delve into the intricacies of geometry, unraveling the key terms, definitions, and theorems that form the foundation of this fascinating subject. Discover the significance of geometry in our world, from its practical applications in architecture and engineering to its role in shaping our understanding of the universe.
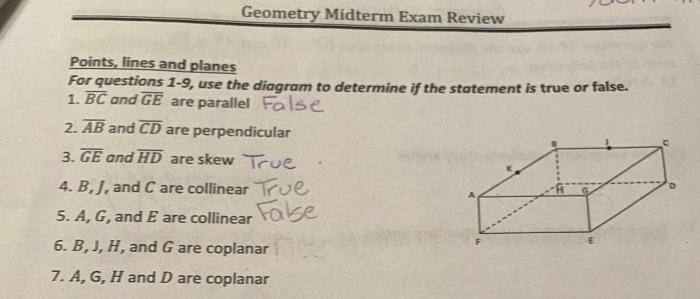
Chapter 3 Geometry Concepts
Chapter 3 of geometry delves into the fundamental concepts that form the foundation of the subject. These concepts provide a framework for understanding the properties of shapes, their relationships, and the measurements associated with them. Understanding these concepts is crucial for building a strong foundation in geometry and for solving more complex problems in higher-level geometry courses.
Key terms and definitions in Chapter 3 include points, lines, planes, angles, triangles, quadrilaterals, circles, and polygons. Points are fundamental building blocks, representing locations in space. Lines are one-dimensional objects that extend infinitely in both directions. Planes are two-dimensional surfaces that extend infinitely in all directions.
Angles are formed by two intersecting lines or rays and are measured in degrees. Triangles, quadrilaterals, circles, and polygons are all specific types of geometric shapes with unique properties and relationships.
Grasping these concepts is essential for comprehending the language of geometry and for developing the ability to analyze and solve geometric problems. These concepts lay the groundwork for understanding more advanced topics in geometry, such as trigonometry, coordinate geometry, and solid geometry.
Chapter Test Questions
Chapter 3 geometry tests typically assess students’ understanding of the fundamental concepts covered in the chapter. Questions may vary in format, including multiple-choice questions, short answer questions, and longer problem-solving questions.
Multiple-choice questions test students’ knowledge of definitions and key terms. Short answer questions require students to explain concepts or provide specific examples. Problem-solving questions present students with a scenario or problem that requires them to apply their understanding of geometry concepts to find a solution.
To effectively solve these types of questions, students should focus on understanding the concepts thoroughly, practicing problem-solving techniques, and developing logical reasoning skills. Reviewing class notes, completing practice problems, and seeking help from teachers or peers can also enhance students’ performance on Chapter 3 geometry tests.
Geometry Problem Solving
Geometry problem solving involves applying logical reasoning and critical thinking to find solutions to geometric problems. The steps involved in solving geometry problems typically include:
- Understanding the problem and identifying the given information
- Drawing a diagram or visual representation to illustrate the problem
- Applying relevant geometry concepts and formulas
- Solving for the unknown variable or quantity
- Checking the solution for reasonableness
Common problem-solving techniques used in geometry include:
- Using properties of shapes and their relationships
- Applying the Pythagorean theorem
- Using coordinate geometry to solve problems involving points and lines
- Using trigonometry to solve problems involving angles and triangles
Developing strong logical reasoning and critical thinking skills is essential for success in geometry problem solving. Students should practice solving a variety of geometry problems to develop their problem-solving abilities.
Quick FAQs: Chapter 3 Chapter Test Geometry Answers
What are the key geometry concepts covered in Chapter 3?
Chapter 3 delves into fundamental concepts such as points, lines, planes, angles, triangles, and polygons, establishing the building blocks of geometry.
How can I effectively solve geometry problems?
Mastering geometry problem-solving involves understanding the problem, drawing diagrams, identifying relevant theorems, and applying logical reasoning to reach a solution.
What are the real-world applications of geometry?
Geometry finds practical applications in architecture, engineering, art, design, and various scientific fields, shaping our built environment and deepening our understanding of the world.