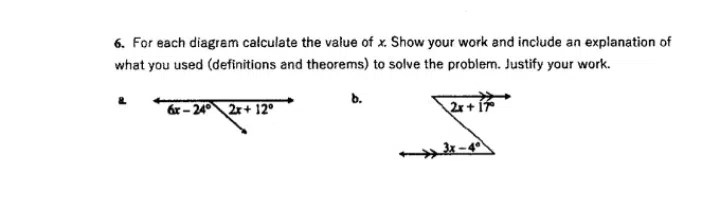
Acp math 53 stewart calc et – Embark on an extraordinary journey into the realm of advanced calculus with ACP Math 53: Stewart Calc ET. Prepare to delve into a comprehensive exploration of fundamental concepts, unlocking the secrets of integration, differential equations, sequences, series, and the captivating world of vectors and matrices.
Throughout this intellectual odyssey, we will unravel the intricacies of Stewart’s Calculus ET textbook, delving into key theorems, principles, and their practical applications in the tapestry of real-world scenarios. Brace yourself for an enlightening adventure that will ignite your passion for the boundless possibilities of mathematics.
Advanced Calculus Concepts in Stewart’s Calculus ET: Acp Math 53 Stewart Calc Et
Stewart’s Calculus ET textbook delves into advanced calculus concepts that extend the foundational principles of calculus, equipping students with a deeper understanding of mathematical analysis. These concepts find applications in various scientific and engineering disciplines, providing a solid mathematical framework for solving complex problems.
Limits and Continuity
Advanced calculus explores the concept of limits and continuity beyond elementary functions. Students learn about indeterminate forms, L’Hôpital’s rule, and the epsilon-delta definition of limits, enabling them to analyze the behavior of functions at specific points and determine their continuity.
Derivatives and Integrals
The study of derivatives and integrals is extended to include techniques for finding derivatives of higher orders, partial derivatives, and multiple integrals. These concepts are essential for understanding the rate of change and accumulation of functions in multivariable settings.
Sequences and Series
Advanced calculus introduces the concept of sequences and series, which are infinite lists of numbers. Students learn about convergence tests, Taylor series, and power series, which provide powerful tools for approximating functions and solving differential equations.
Vector Calculus
Vector calculus extends the concepts of calculus to multidimensional space, introducing vectors, vector fields, and line integrals. These concepts are crucial for understanding the flow of fluids, the behavior of electromagnetic fields, and the motion of objects in three-dimensional space.
Applications
Throughout the textbook, Stewart emphasizes the relevance of advanced calculus concepts in real-world applications. Examples include using derivatives to optimize functions, integrals to calculate volumes and areas, and vector calculus to model physical phenomena such as fluid dynamics and electromagnetism.
Techniques and Applications of Integration
Integration is a fundamental concept in calculus that allows us to find the area under a curve, the volume of a solid, and many other important quantities. There are a number of different techniques for integrating functions, and the choice of technique depends on the function being integrated.
Some of the most common integration techniques include:
- Substitution
- Integration by parts
- Trigonometric substitution
Each of these techniques has its own advantages and disadvantages, and the best technique to use will depend on the specific function being integrated.
Substitution
Substitution is a technique that can be used to integrate functions that are composed of other functions. For example, to integrate the function f(x) = x2+ 1 , we can use the substitution u = x2+ 1 . This gives us du = 2x dx, and we can rewrite the integral as:
“`∫(x 2+ 1) dx = ∫u 1/2(du/2) = (1/2)∫u 1/2du = (1/2)(2/3)u 3/2+ C = (1/3)(x 2+ 1) 3/2+ C“`
Integration by Parts
Integration by parts is a technique that can be used to integrate functions that are the product of two other functions. For example, to integrate the function f(x) = x sin(x), we can use the integration by parts formula:
“`∫u dv = uv
If you’re tackling ACP Math 53 Stewart Calc ET and need a break, why not try the zig and zag crossword clue ? It’s a fun way to exercise your brain in a different way. Once you’ve solved that, come back refreshed and ready to conquer the rest of ACP Math 53 Stewart Calc ET.
∫v du
“`
where uand vare two functions of x. In this case, we can let u = xand dv = sin(x) dx. This gives us du = dxand v =-cos(x) , and we can rewrite the integral as:
“`∫x sin(x) dx = x(-cos(x))
- ∫(-cos(x)) dx =
- x cos(x) + sin(x) + C
“`
Trigonometric Substitution, Acp math 53 stewart calc et
Trigonometric substitution is a technique that can be used to integrate functions that involve trigonometric functions. For example, to integrate the function f(x) = 1/sqrt(1- x 2) , we can use the trigonometric substitution x = sin(θ). This gives us dx = cos(θ) dθ, and we can rewrite the integral as:
“`∫1/sqrt(1
x2) dx = ∫1/cos(θ) cos(θ) dθ = ∫1 dθ = θ + C = sin -1(x) + C
“`
Applications of Integration
Integration has a wide range of applications in various fields, including:
- Physics: Integration can be used to find the area under a velocity-time graph, which gives the distance traveled by an object.
- Engineering: Integration can be used to find the volume of a solid, the surface area of a surface, and the work done by a force.
- Economics: Integration can be used to find the total revenue, the total cost, and the consumer surplus.
Differential Equations and Their Applications
Differential equations are mathematical equations that describe the relationship between a function and its derivatives. They are used to model a wide variety of real-world phenomena, including population growth, radioactive decay, and the motion of objects.
Types of Differential Equations
There are many different types of differential equations, but the most common are:
- Ordinary differential equations (ODEs)involve only one independent variable, typically time.
- Partial differential equations (PDEs)involve more than one independent variable, typically space and time.
- Linear differential equationshave coefficients that are constants or functions of the independent variable only.
- Nonlinear differential equationshave coefficients that are functions of the dependent variable or its derivatives.
Methods for Solving Differential Equations
There are a variety of methods for solving differential equations, including:
- Separation of variables
- Laplace transforms
- Numerical methods
Applications of Differential Equations
Differential equations are used to model a wide variety of real-world phenomena, including:
- Population growth
- Radioactive decay
- The motion of objects
- Fluid dynamics
- Heat transfer
Sequences and Series
Sequences and series are fundamental concepts in mathematics that involve the study of ordered lists of numbers. They play a crucial role in various fields, including calculus, probability, and statistics.
A sequence is an ordered list of numbers, where each number is called a term. The terms are typically denoted by a1, a2, a3, …, and so on. A series is the sum of the terms of a sequence. It is denoted by the notation ∑n=1^∞ an.
Convergence and Divergence Tests for Series
One of the key concepts in the study of series is convergence and divergence. A series is said to be convergent if the sum of its terms approaches a finite value as the number of terms approaches infinity. Otherwise, the series is said to be divergent.
There are several tests that can be used to determine whether a series converges or diverges. Some of the most commonly used tests include:
- The Integral Test
- The Comparison Test
- The Limit Comparison Test
- The Ratio Test
- The Root Test
Applications of Sequences and Series
Sequences and series have a wide range of applications in various fields. Some of the most notable applications include:
- Probability:Sequences and series are used to model the probability of events occurring. For example, the Poisson distribution is a probability distribution that describes the number of events occurring in a fixed interval of time or space.
- Calculus:Sequences and series are used to define and evaluate limits, derivatives, and integrals. For example, the Taylor series is a power series that can be used to approximate the value of a function at a given point.
Vectors and Matrices in Calculus
Vectors and matrices are mathematical tools that play a significant role in calculus. They provide a way to represent and manipulate multi-dimensional data, which is essential for solving problems in areas such as linear algebra and differential equations.
Vectors
- A vector is a quantity that has both magnitude and direction. It can be represented geometrically as an arrow, with the length of the arrow representing the magnitude and the direction of the arrow representing the direction.
- Vectors are often used to represent forces, velocities, and other physical quantities that have both magnitude and direction.
- Vectors can be added and subtracted using the parallelogram law. The sum of two vectors is the vector that forms the diagonal of the parallelogram with the two vectors as sides.
- Vectors can also be multiplied by scalars, which are numbers. The product of a vector and a scalar is a vector that has the same direction as the original vector, but with a magnitude that is scaled by the scalar.
Matrices
- A matrix is a rectangular array of numbers. It can be used to represent a system of linear equations, a transformation, or a set of data.
- Matrices can be added and subtracted by adding or subtracting the corresponding elements. They can also be multiplied by scalars by multiplying each element by the scalar.
- Matrices can be multiplied by other matrices to produce a new matrix. The product of two matrices is a matrix whose elements are the sums of the products of the corresponding elements of the original matrices.
- Matrices are used in a wide variety of applications, including solving systems of linear equations, finding eigenvalues and eigenvectors, and representing transformations.
Commonly Asked Questions
What is the significance of ACP Math 53: Stewart Calc ET?
This course provides a comprehensive foundation in advanced calculus, equipping students with the knowledge and skills essential for further studies and careers in STEM fields.
How does this course differ from other calculus courses?
ACP Math 53: Stewart Calc ET delves deeper into advanced concepts, exploring topics such as differential equations, sequences, series, and the applications of vectors and matrices in calculus.
What are the prerequisites for ACP Math 53: Stewart Calc ET?
A strong foundation in introductory calculus, including concepts of limits, derivatives, and integrals, is essential.